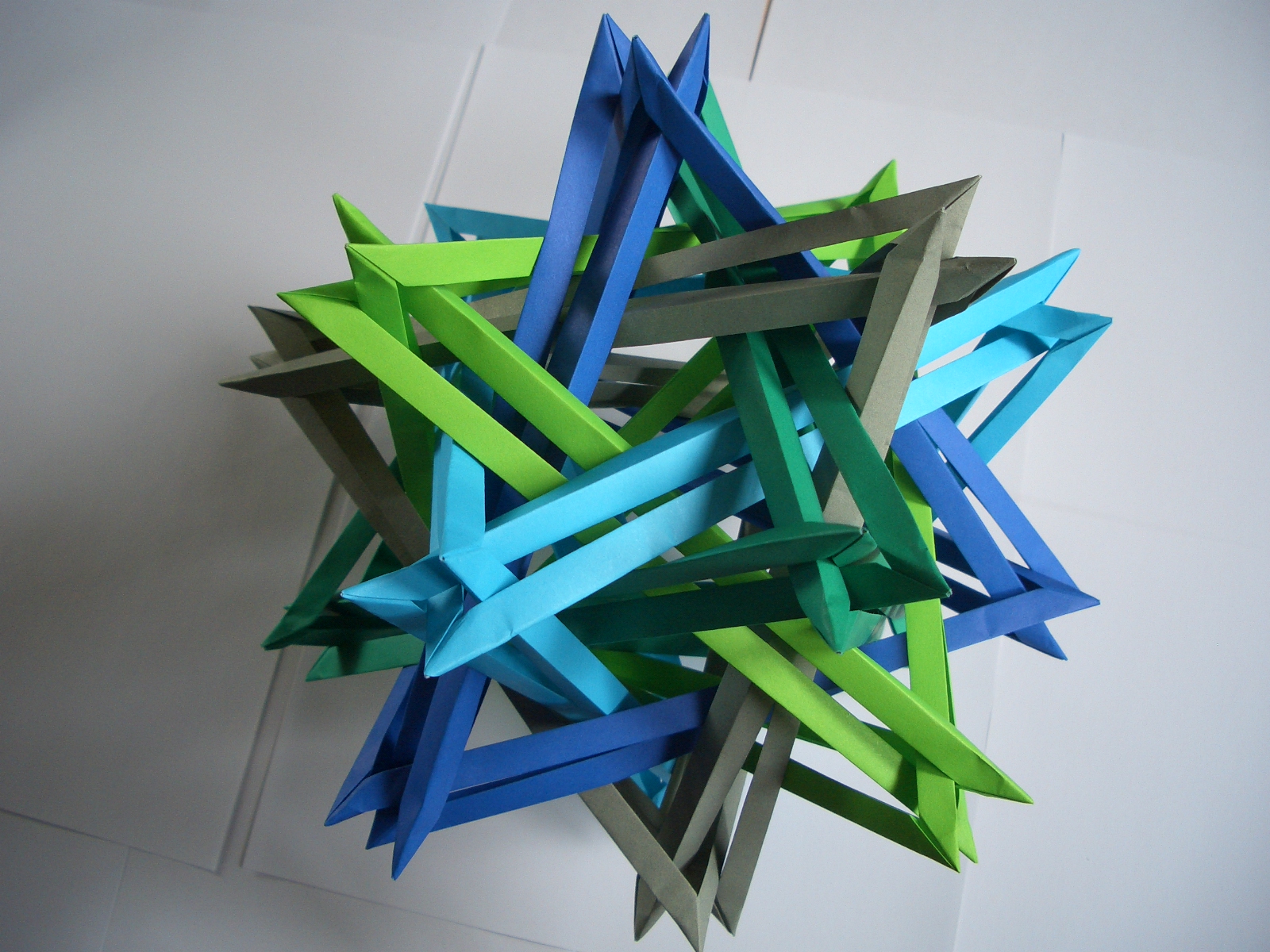
This is one of the most famous polypolyhdra found by Robert J. Lang. It is made of 60 edges in 20 triangles. There are 5 sub structures that are interfused. Each consists of 4 triangles that make a tetrahedra-like compound. This model is exceptionally hard to assemble and therefor called “K2” after the mountain track up the mount everest.
For me, its beauty lies in the simplicity of the modules arranged in a complex spiky yet structered way.
The folding ins tructions for the modules are quite easy but needs time. You need several hours to prepare all 60 modules and roughly the same amount of time to assemble the model. It took me around 8 hours with some reacreation breaks. There are professional instructions of the creator available in a book, but as I have not yet acquired it, I simply used a technique I already knew. The Francis Ow 60° unit gives a nice 60 dregree angle for the ends of each module. It is most commonly used to build the “five intersecting tetrahedra”. But for the K2 you need a length/width-ratio of 6. So you cut 1 square paper into 6 rectangles and fold the Francis Ow sequence on each of them. Now for the assembly process I had help that came in form of a 3D-representation of the model in the web browser that you can rotate freely. It was created by Carlos Furuti.
tructions for the modules are quite easy but needs time. You need several hours to prepare all 60 modules and roughly the same amount of time to assemble the model. It took me around 8 hours with some reacreation breaks. There are professional instructions of the creator available in a book, but as I have not yet acquired it, I simply used a technique I already knew. The Francis Ow 60° unit gives a nice 60 dregree angle for the ends of each module. It is most commonly used to build the “five intersecting tetrahedra”. But for the K2 you need a length/width-ratio of 6. So you cut 1 square paper into 6 rectangles and fold the Francis Ow sequence on each of them. Now for the assembly process I had help that came in form of a 3D-representation of the model in the web browser that you can rotate freely. It was created by Carlos Furuti.
For my second K2 I made some pictures during the assembly. Here you can see that I temporarily used rubber bands to hold everything in place. This way keeps the frustration level at a minimum and you can enjoy putting the model together without worrying that the triangles will fall into chaos.
For those who are interessted in the elegant mathematic background of polypolyhedra I suggest you read the original article on polypolyhedra from Robert J. Lang along with his scientific papers he wrote about the matter.
Here you can find all the ressources:
- original article on polypolyhedra (langorigami.com)
- Francis Ow 60° unit
- 3D-model in web browser (Carlos Furuti)